Algebra can be a daunting subject for many students, but with the right approach, it can be simplified and made more accessible. One of the most fundamental concepts in algebra is solving equations, and in this article, we will focus on solving 2x + 3x equations.
Solving equations is a crucial skill in algebra, as it allows us to find the value of unknown variables. In this article, we will break down the steps to solve 2x + 3x equations, provide examples, and offer tips to help you master this skill.
Why is Solving Equations Important?
Solving equations is essential in algebra because it helps us to:
- Find the value of unknown variables
- Check the validity of mathematical statements
- Solve problems in various fields, such as physics, engineering, and economics
Understanding the Basics of Equations
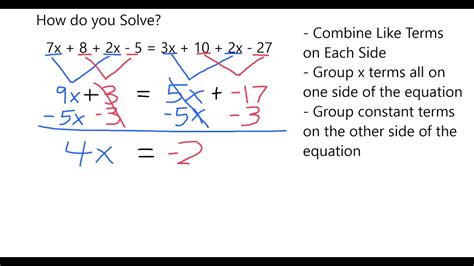
Before we dive into solving 2x + 3x equations, let's review the basics of equations. An equation is a mathematical statement that expresses the equality of two expressions, typically containing variables and constants. The goal of solving an equation is to isolate the variable on one side of the equation.
For example, consider the equation 2x + 3 = 5. To solve for x, we need to isolate x on one side of the equation.
Solving 2x + 3x Equations
Now, let's focus on solving 2x + 3x equations. These equations can be written in the form:
2x + 3x = constant
To solve these equations, we can follow these steps:
- Combine like terms: Combine the x terms on the left side of the equation.
- Isolate x: Use addition, subtraction, multiplication, or division to isolate x on one side of the equation.
Let's consider an example:
2x + 3x = 12
Step 1: Combine like terms
Combine the x terms on the left side of the equation:
5x = 12
Step 2: Isolate x
Divide both sides of the equation by 5 to isolate x:
x = 12/5
x = 2.4
Therefore, the solution to the equation 2x + 3x = 12 is x = 2.4.
Tips and Tricks
Here are some tips and tricks to help you solve 2x + 3x equations:
- Always combine like terms before isolating the variable.
- Use inverse operations to isolate the variable. For example, if the equation contains a + symbol, use subtraction to isolate the variable.
- Check your work by plugging the solution back into the original equation.

Real-World Applications
Solving 2x + 3x equations has numerous real-world applications. For example:
- Physics: Solving equations is crucial in physics to describe the motion of objects, forces, and energies.
- Engineering: Equations are used to design and optimize systems, such as bridges, buildings, and electronic circuits.
- Economics: Equations are used to model economic systems, understand the behavior of markets, and make predictions.
Conclusion
Solving 2x + 3x equations is a fundamental skill in algebra that requires attention to detail and practice. By following the steps outlined in this article, you can master this skill and apply it to various real-world applications. Remember to always combine like terms, isolate the variable, and check your work.
Gallery of Algebra Solutions





FAQs
What is the difference between an equation and an expression?
+An equation is a mathematical statement that expresses the equality of two expressions, while an expression is a collection of numbers, variables, and mathematical operations.
How do I solve an equation with multiple variables?
+To solve an equation with multiple variables, you need to isolate one variable at a time. Use substitution or elimination methods to solve for one variable, and then substitute that value into the original equation to solve for the other variable.
What is the purpose of solving equations?
+The purpose of solving equations is to find the value of unknown variables, which can be used to describe real-world phenomena, make predictions, and solve problems in various fields.
Call to Action
We hope this article has helped you understand the basics of solving 2x + 3x equations. Practice makes perfect, so try solving some equations on your own and check your work. If you have any questions or need further clarification, feel free to ask in the comments section below.