In the realm of statistics, few concepts hold as much significance as population variance. This fundamental concept plays a pivotal role in understanding the nature of data distribution, and its implications resonate across various fields, including economics, social sciences, and natural sciences. Despite its importance, population variance often remains a mysterious entity, hiding in plain sight. In this article, we aim to demystify the concept of population variance, exploring its definition, importance, and applications.

Defining Population Variance
Population variance refers to the average of the squared differences between individual data points and the mean of the population. It provides a measure of the dispersion or spread of data within a population. In other words, population variance quantifies the degree to which data points deviate from the mean value.
Understanding the Formula
The population variance formula is given by:
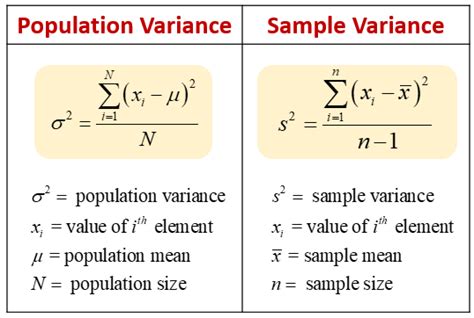
σ² = Σ(xi - μ)² / N
where:
- σ² represents the population variance
- xi denotes the individual data points
- μ represents the mean of the population
- N denotes the total number of data points
This formula calculates the average of the squared differences between each data point and the mean, providing a comprehensive understanding of the data's dispersion.
The Importance of Population Variance
Population variance plays a vital role in various statistical applications, including:
- Hypothesis Testing: Population variance is used to test hypotheses about the population mean, allowing researchers to draw conclusions about the population based on sample data.
- Confidence Intervals: By estimating population variance, researchers can construct confidence intervals to quantify the uncertainty associated with sample statistics.
- Regression Analysis: Population variance is used to estimate the variability in the residuals of regression models, enabling researchers to evaluate the model's goodness of fit.

Real-World Applications
Population variance has far-reaching implications in various fields, including:
- Finance: Understanding population variance helps investors estimate the potential risks associated with different investment portfolios.
- Economics: Population variance is used to analyze the distribution of income and wealth, enabling policymakers to develop targeted economic policies.
- Social Sciences: Researchers use population variance to study the dispersion of social phenomena, such as crime rates or education levels.
How to Calculate Population Variance
Calculating population variance involves several steps:
- Collect Data: Gather a representative sample of data from the population.
- Calculate the Mean: Compute the mean of the sample data.
- Calculate the Squared Differences: Calculate the squared differences between each data point and the mean.
- Calculate the Average: Calculate the average of the squared differences.
- Estimate Population Variance: Use the sample data to estimate the population variance.

Common Misconceptions
- Sample Variance vs. Population Variance: Many researchers mistakenly use sample variance as a substitute for population variance. However, sample variance is only an estimate of population variance.
- Assuming Normality: Population variance assumes normality, but real-world data often deviates from normality. It is essential to test for normality before using population variance.

Conclusion: Unlocking the True Value of Population Variance
Population variance is a fundamental concept in statistics that provides valuable insights into data dispersion. By understanding the definition, importance, and applications of population variance, researchers can unlock its true value. Whether in finance, economics, or social sciences, population variance plays a crucial role in data analysis and decision-making. As we continue to navigate the complexities of data-driven decision-making, it is essential to recognize the significance of population variance and harness its power to drive meaningful insights.






What is population variance?
+Population variance refers to the average of the squared differences between individual data points and the mean of the population.
Why is population variance important?
+Population variance plays a crucial role in various statistical applications, including hypothesis testing, confidence intervals, and regression analysis.
How do I calculate population variance?
+Calculating population variance involves collecting data, calculating the mean, calculating the squared differences, and estimating population variance using the sample data.
We hope this article has provided you with a comprehensive understanding of population variance. If you have any further questions or would like to share your thoughts, please feel free to comment below.