Equivalent Expressions: Simplifying Math With Ease

Quick Links :

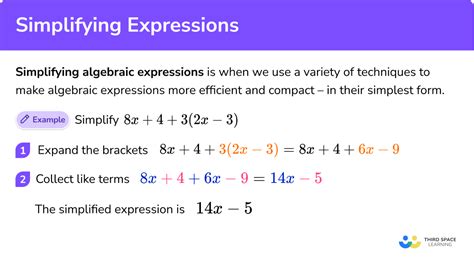
The world of mathematics can be a complex and daunting place, especially when dealing with expressions that seem to go on forever. However, with the right tools and techniques, simplifying math can be a breeze. One such technique is using equivalent expressions, which can help you simplify even the most complicated math problems with ease.

What Are Equivalent Expressions?

Equivalent expressions are mathematical expressions that have the same value or represent the same relationship, but are written in different ways. For example, the expressions 2(3) and 6 are equivalent because they both equal 6. Similarly, the expressions x + 2 and 2 + x are equivalent because they both represent the same relationship between the variables.

Why Are Equivalent Expressions Important?

Equivalent expressions are important because they can help you simplify complex math problems by rewriting them in a more manageable form. By recognizing equivalent expressions, you can often simplify problems by:

• Combining like terms
• Canceling out common factors
• Rearranging the order of operations
• Substituting equivalent expressions into a problem

How to Identify Equivalent Expressions

Identifying equivalent expressions can be a bit tricky, but there are some strategies you can use to make it easier. Here are a few tips:

• Look for common factors: If two expressions have a common factor, you can often simplify them by canceling out that factor.
• Check for commutative properties: The commutative properties of addition and multiplication state that the order of the numbers does not change the result. For example, 2 + 3 is equivalent to 3 + 2.
• Use the distributive property: The distributive property states that a single operation can be applied to multiple numbers. For example, 2(x + 3) is equivalent to 2x + 6.

Examples of Equivalent Expressions

Here are a few examples of equivalent expressions:

• 2x + 5 and x + 2x + 5
• 3(2x) and 6x
• x^2 + 4x + 4 and (x + 2)(x + 2)

Using Equivalent Expressions to Simplify Math Problems

Now that you know how to identify equivalent expressions, let's see how you can use them to simplify math problems.

Example 1: Simplifying an Algebraic Expression

Suppose you have the algebraic expression 2x + 5 + 3x. You can simplify this expression by combining like terms:

2x + 5 + 3x = 5x + 5

Example 2: Simplifying a Fraction

Suppose you have the fraction 4/8. You can simplify this fraction by canceling out the common factor of 4:

4/8 = 1/2

Conclusion: Equivalent Expressions Make Math Easier

Equivalent expressions are a powerful tool for simplifying math problems. By recognizing equivalent expressions, you can often simplify complex problems by combining like terms, canceling out common factors, rearranging the order of operations, and substituting equivalent expressions into a problem. With practice and patience, you can become proficient in using equivalent expressions to make math easier.

Take Action: Practice Using Equivalent Expressions

Try practicing using equivalent expressions to simplify math problems. You can find plenty of examples online or in math textbooks. With practice, you'll become more comfortable using equivalent expressions to simplify even the most complicated math problems.