The Fahrenheit to Celsius equation is a fundamental concept in physics and engineering, used to convert temperatures from one unit to another. The equation is simple, yet powerful, and is widely used in various fields, including science, technology, engineering, and mathematics (STEM). In this article, we will delve into the world of temperature conversions and explore the slope of the Fahrenheit to Celsius equation.
What is the Fahrenheit to Celsius Equation?
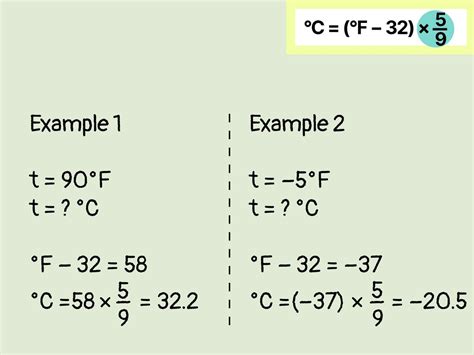
The Fahrenheit to Celsius equation is a linear equation that relates the temperature in Fahrenheit (°F) to the temperature in Celsius (°C). The equation is:
°C = (°F - 32) × 5/9
This equation can be used to convert any temperature from Fahrenheit to Celsius.
Understanding the Slope of the Equation
The slope of the Fahrenheit to Celsius equation is a crucial concept that helps us understand the relationship between the two temperature scales. The slope represents the rate of change of the temperature in Celsius with respect to the temperature in Fahrenheit.
To calculate the slope, we can rearrange the equation to isolate the temperature in Celsius:
°C = (5/9) × (°F - 32)
The slope of this equation is 5/9, which represents the rate of change of the temperature in Celsius per unit change in the temperature in Fahrenheit.
Interpreting the Slope
The slope of the Fahrenheit to Celsius equation has a significant impact on our understanding of temperature conversions. A slope of 5/9 means that for every 1-degree change in Fahrenheit, the temperature in Celsius changes by 5/9 degrees.
For example, if the temperature in Fahrenheit increases by 10 degrees, the temperature in Celsius will increase by (5/9) × 10 = 5.56 degrees. This means that the temperature in Celsius changes at a slower rate than the temperature in Fahrenheit.
Practical Applications of the Slope
The slope of the Fahrenheit to Celsius equation has numerous practical applications in various fields. Here are a few examples:
- Temperature Conversion: The slope of the equation is essential for converting temperatures from Fahrenheit to Celsius. By understanding the slope, we can quickly and accurately convert temperatures between the two scales.
- Temperature Calibration: The slope of the equation is also crucial for calibrating thermometers and temperature sensors. By knowing the slope, we can adjust the calibration curve to ensure accurate temperature readings.
- Heat Transfer: The slope of the equation plays a significant role in heat transfer calculations. By understanding the slope, we can calculate the heat transfer rates between different systems and materials.
Gallery of Temperature Conversion Charts






Frequently Asked Questions
What is the Fahrenheit to Celsius equation?
+The Fahrenheit to Celsius equation is a linear equation that relates the temperature in Fahrenheit (°F) to the temperature in Celsius (°C). The equation is: °C = (°F - 32) × 5/9
What is the slope of the Fahrenheit to Celsius equation?
+The slope of the Fahrenheit to Celsius equation is 5/9, which represents the rate of change of the temperature in Celsius per unit change in the temperature in Fahrenheit.
How is the slope of the equation used in practical applications?
+The slope of the equation is used in various practical applications, including temperature conversion, temperature calibration, and heat transfer calculations.
In conclusion, the slope of the Fahrenheit to Celsius equation is a fundamental concept that plays a significant role in our understanding of temperature conversions. By understanding the slope, we can quickly and accurately convert temperatures between the two scales, and apply this knowledge in various practical applications.