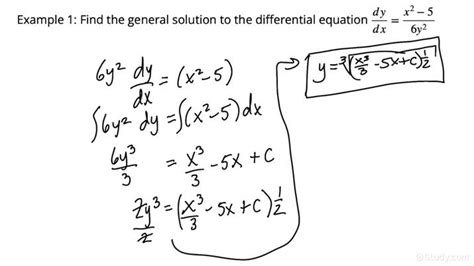
Differential equations are a fundamental tool in mathematics and have numerous applications in various fields, including physics, engineering, economics, and biology. These equations describe how quantities change over time or space, and they are essential in modeling real-world problems. In this article, we will explore five ways differential equations solve real-world problems, providing practical examples and illustrating the significance of these equations in different fields.
Differential equations have been widely used to model and analyze various phenomena, from the motion of objects to the spread of diseases. They provide a powerful tool for understanding complex systems and making predictions about their behavior. By solving differential equations, we can gain valuable insights into the underlying mechanisms of real-world problems and develop effective solutions.
Understanding Population Growth and Spread of Diseases
One of the most significant applications of differential equations is in the field of epidemiology, where they are used to model the spread of diseases. The SIR model, which describes the spread of infectious diseases, is a classic example of a differential equation in action. This model divides the population into three compartments: susceptible, infected, and recovered. By solving the differential equations that govern the SIR model, researchers can predict the spread of diseases and develop strategies for controlling outbreaks.
For instance, during the COVID-19 pandemic, differential equations were used to model the spread of the virus and predict the number of cases and hospitalizations. This information was crucial for policymakers to make informed decisions about lockdowns, mask mandates, and vaccination strategies.

Modeling Electrical Circuits and Electronic Devices
Differential equations are also used extensively in electrical engineering to model and analyze electrical circuits and electronic devices. The behavior of these circuits and devices can be described using differential equations, which are then solved to determine the voltage, current, and other relevant quantities.
For example, the behavior of a simple RC circuit can be modeled using a differential equation that describes how the voltage across the capacitor changes over time. By solving this equation, engineers can design and optimize electronic devices, such as filters and amplifiers.

Predicting Stock Prices and Portfolio Optimization
Differential equations have also been applied in finance to model and predict stock prices. The Black-Scholes model, which describes the behavior of stock prices, is a well-known example of a differential equation in finance. This model assumes that the stock price follows a geometric Brownian motion, and the differential equation that governs this motion is solved to determine the price of options and other derivatives.
In addition, differential equations can be used to optimize portfolios by minimizing risk and maximizing returns. By solving these equations, investors can determine the optimal allocation of assets in their portfolios and make informed decisions about their investments.

Analyzing and Modeling Traffic Flow
Differential equations can also be used to model and analyze traffic flow, which is a complex phenomenon that involves the interaction of many vehicles and drivers. The Lighthill-Whitham-Richards (LWR) model, which describes the behavior of traffic flow, is a classic example of a differential equation in action. This model assumes that the traffic flow is governed by a set of differential equations that describe the conservation of cars and the flow of traffic.
By solving these equations, researchers can gain insights into the behavior of traffic flow and develop strategies for optimizing traffic flow and reducing congestion.

Optimizing Control Systems and Robotics
Finally, differential equations are used extensively in control theory to optimize control systems and robotics. The behavior of these systems can be described using differential equations, which are then solved to determine the optimal control strategy.
For example, the behavior of a robotic arm can be modeled using a set of differential equations that describe the motion of the arm. By solving these equations, engineers can develop control strategies that optimize the motion of the arm and achieve precise control.

Gallery of Differential Equations in Action






FAQs
What is a differential equation?
+A differential equation is a mathematical equation that describes how a quantity changes over time or space.
What are some common applications of differential equations?
+Differential equations have numerous applications in physics, engineering, economics, and biology, including modeling population growth, optimizing control systems, and predicting stock prices.
How are differential equations solved?
+Differential equations can be solved using various methods, including analytical and numerical methods, such as separation of variables, integration, and numerical integration.
In conclusion, differential equations play a vital role in solving real-world problems across various fields. By modeling and analyzing complex phenomena using differential equations, researchers and practitioners can gain valuable insights and develop effective solutions. Whether it's predicting stock prices, optimizing control systems, or modeling population growth, differential equations are an essential tool in understanding and addressing the challenges of our world.