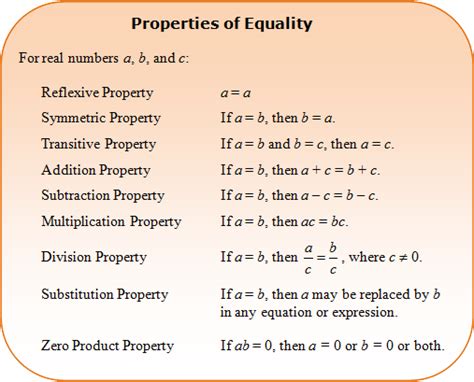
Math Properties For Solving Equations Easily

Quick Links :

Math properties are fundamental concepts in mathematics that help us solve equations and manipulate expressions in a consistent and predictable way. Understanding these properties is essential for anyone who wants to improve their math skills and solve equations with ease. In this article, we will explore the different types of math properties, their definitions, and examples of how to use them to solve equations.

What are Math Properties?

Math properties are rules or laws that govern how numbers and mathematical operations interact with each other. They are used to describe the behavior of mathematical expressions and equations, and they provide a framework for solving equations and manipulating expressions in a logical and consistent way.

Types of Math Properties

There are several types of math properties, including:

• Commutative Property
• Associative Property
• Distributive Property
• Identity Property
• Inverse Property

Each of these properties has its own definition and examples, which we will explore in more detail below.

Commutative Property

The commutative property states that the order of the numbers or variables in an equation does not change the result. In other words, if we have two numbers or variables, a and b, the equation a + b = b + a is always true.

For example, if we have the equation 2 + 3 =?, we can use the commutative property to rewrite the equation as 3 + 2 =?, which is also equal to 5.

Associative Property

The associative property states that when we have three or more numbers or variables in an equation, the order in which we perform the operations does not change the result. In other words, if we have three numbers or variables, a, b, and c, the equation (a + b) + c = a + (b + c) is always true.

For example, if we have the equation (2 + 3) + 4 =?, we can use the associative property to rewrite the equation as 2 + (3 + 4) =?, which is also equal to 9.

Distributive Property

The distributive property states that when we have two or more numbers or variables in an equation, we can distribute one of the numbers or variables to the others. In other words, if we have two numbers or variables, a and b, and a third number or variable, c, the equation a(b + c) = ab + ac is always true.

For example, if we have the equation 2(3 + 4) =?, we can use the distributive property to rewrite the equation as 2(3) + 2(4) =?, which is also equal to 14.

Identity Property

The identity property states that when we have a number or variable in an equation, we can add or multiply it by a special number, called the identity element, without changing the result. In other words, if we have a number or variable, a, the equation a + 0 = a and a × 1 = a are always true.

For example, if we have the equation 2 + 0 =?, we can use the identity property to rewrite the equation as 2 =?, which is also equal to 2.

Inverse Property

The inverse property states that when we have a number or variable in an equation, we can add or multiply it by a special number, called the inverse element, to get the opposite result. In other words, if we have a number or variable, a, the equation a + (-a) = 0 and a × (1/a) = 1 are always true.

For example, if we have the equation 2 + (-2) =?, we can use the inverse property to rewrite the equation as 0 =?, which is also equal to 0.

Gallery of Math Properties

FAQs

In conclusion, math properties are essential concepts in mathematics that help us solve equations and manipulate expressions in a consistent and predictable way. By understanding the different types of math properties, including the commutative, associative, distributive, identity, and inverse properties, we can improve our math skills and solve equations with ease.