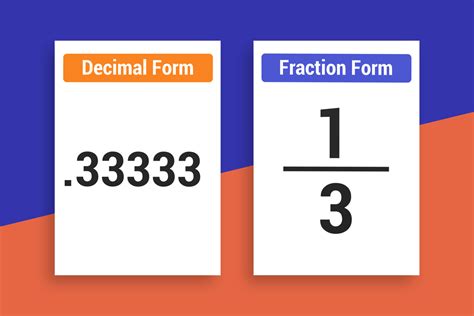
Converting a repeating decimal to a fraction can be a useful skill in mathematics and science. One common repeating decimal is 0.33333, which is equal to one-third. Here are three ways to convert 0.33333 to a fraction.
0.33333 is a repeating decimal, meaning that the digit 3 repeats indefinitely. This can be denoted by a bar over the repeating digit, like this: 0.3̄.

Method 1: Algebraic Method
One way to convert 0.33333 to a fraction is to use algebra. Let's say that x = 0.33333. We can multiply both sides of the equation by 10 to get 10x = 3.33333.

Now, we can subtract the original equation from the new equation to get 10x - x = 3.33333 - 0.33333. This simplifies to 9x = 3, which means that x = 3/9 or 1/3.
Method 2: Geometric Method
Another way to convert 0.33333 to a fraction is to use geometry. We can represent the repeating decimal as a geometric series, like this: 0.33333 = 3/10 + 3/100 + 3/1000 +...

We can use the formula for the sum of an infinite geometric series to find the value of this series. The formula is S = a / (1 - r), where a is the first term and r is the common ratio. In this case, a = 3/10 and r = 1/10.
Plugging in these values, we get S = (3/10) / (1 - 1/10) = (3/10) / (9/10) = 3/9 or 1/3.
Method 3: Division Method
A third way to convert 0.33333 to a fraction is to use division. We can divide 0.33333 by 1 to get 0.33333 ÷ 1 = 1/3.

This method is quick and easy, but it may not work for all repeating decimals.
Comparison of Methods
All three methods produce the same result: 0.33333 is equal to 1/3. However, the algebraic method is often the most useful, as it can be applied to any repeating decimal.

In conclusion, there are several ways to convert 0.33333 to a fraction. The algebraic method is often the most useful, but the geometric and division methods can also be applied in certain situations.






We hope this article has helped you understand how to convert 0.33333 to a fraction. If you have any questions or need further clarification, please don't hesitate to ask.
What is a repeating decimal?
+A repeating decimal is a decimal number that has a block of digits that repeats indefinitely.
How can I convert a repeating decimal to a fraction?
+There are several ways to convert a repeating decimal to a fraction, including the algebraic method, geometric method, and division method.
What is the algebraic method for converting a repeating decimal to a fraction?
+The algebraic method involves multiplying the repeating decimal by a power of 10 to shift the repeating block, then subtracting the original decimal from the new decimal to eliminate the repeating block.