5 Ways To Apply Slope Intercept Form

Quick Links :

The slope-intercept form is a fundamental concept in algebra and is used to graph linear equations. It's a powerful tool that can be applied in various real-world situations, from physics and engineering to economics and computer science. In this article, we'll explore five ways to apply the slope-intercept form and demonstrate its versatility.

Understanding the Slope-Intercept Form

Before we dive into the applications, let's quickly review the slope-intercept form. The slope-intercept form of a linear equation is given by:

y = mx + b

where:

• m is the slope of the line
• b is the y-intercept (the point where the line crosses the y-axis)
• x is the independent variable
• y is the dependent variable

1. Graphing Linear Equations

One of the most common applications of the slope-intercept form is graphing linear equations. By using the slope and y-intercept, we can easily plot the line on a coordinate plane.

For example, let's graph the equation y = 2x + 3. We can start by plotting the y-intercept (0, 3) and then use the slope (2) to find another point on the line.

2. Finding the Equation of a Line

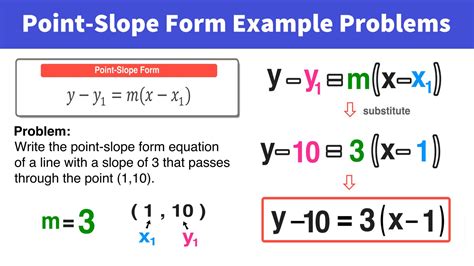
Another application of the slope-intercept form is finding the equation of a line given two points. We can use the slope formula to find the slope of the line and then use the point-slope form to find the equation.

For example, let's find the equation of the line that passes through the points (2, 3) and (4, 5). We can use the slope formula to find the slope:

m = (y2 - y1) / (x2 - x1) = (5 - 3) / (4 - 2) = 2 / 2 = 1

Then, we can use the point-slope form to find the equation:

y - y1 = m(x - x1) y - 3 = 1(x - 2) y = x + 1

3. Predicting Linear Relationships

The slope-intercept form can also be used to predict linear relationships between variables. For example, let's say we're analyzing the relationship between the amount of money spent on advertising and the number of sales.

We can use the slope-intercept form to create a linear model that predicts the number of sales based on the amount of money spent on advertising. Let's say our data shows that for every dollar spent on advertising, sales increase by 2 units. We can use this information to create a linear equation:

Sales = 2(Advertising) + b

We can then use this equation to predict sales based on the amount of money spent on advertising.

4. Optimizing Linear Functions

The slope-intercept form can also be used to optimize linear functions. For example, let's say we're trying to maximize the profit of a company that produces two products, A and B.

We can use the slope-intercept form to create a linear function that represents the profit of the company:

Profit = 2x + 3y

where x is the number of units of product A produced and y is the number of units of product B produced.

We can then use this equation to optimize the production levels of the two products to maximize profit.

5. Analyzing Linear Systems

Finally, the slope-intercept form can be used to analyze linear systems. For example, let's say we're analyzing a system of two linear equations:

2x + 3y = 7 x - 2y = -3

We can use the slope-intercept form to rewrite the two equations:

y = -2/3x + 7/3 y = 1/2x - 3/2

We can then use these equations to analyze the system and find the solution.

Gallery of Slope-Intercept Form Applications

Frequently Asked Questions

We hope this article has demonstrated the versatility and importance of the slope-intercept form. Whether you're graphing linear equations, finding the equation of a line, predicting linear relationships, optimizing linear functions, or analyzing linear systems, the slope-intercept form is a powerful tool that can help you achieve your goals.