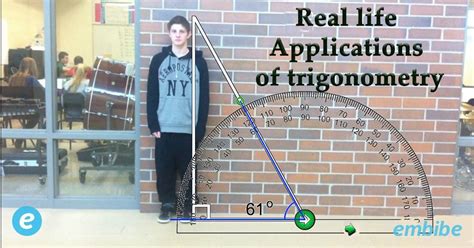
The field of mathematics is vast and encompasses various branches, each with its unique applications in real-life scenarios. Trigonometry, in particular, is one such branch that has numerous practical applications across different industries. While many of us may think of trigonometry as a mere mathematical concept, its significance in real-life applications cannot be overstated. From navigation and physics to engineering and computer science, trigonometry plays a vital role in solving complex problems and making our lives easier.
In this article, we will delve into five real-life applications of trigonometry, exploring how this branch of mathematics impacts various aspects of our daily lives.
1. Navigation and Surveying
Trigonometry has been an essential tool for navigation and surveying for centuries. The concept of triangulation, which involves using trigonometric functions to determine the distance between two points, is widely used in navigation systems such as GPS. By using the angles and sides of triangles, GPS systems can accurately determine the location of a vehicle or device, making navigation easier and more efficient.
In surveying, trigonometry is used to calculate distances and angles between landmarks and reference points. This information is crucial in determining property boundaries, building layouts, and mapping terrain. Surveyors use trigonometric functions to calculate the height of buildings, the depth of excavations, and the distance between reference points.
**

2. Physics and Engineering
Trigonometry is a fundamental concept in physics and engineering, particularly in the study of waves and oscillations. The trigonometric functions sine, cosine, and tangent are used to describe the behavior of waves, such as sound waves, light waves, and water waves.
In engineering, trigonometry is used to calculate the stress and strain on buildings, bridges, and other structures. By analyzing the angles and forces acting on a structure, engineers can determine the maximum weight it can support and the stress it can withstand.
**

3. Computer Science and Graphics
Trigonometry is a crucial component of computer science, particularly in the field of computer graphics. The trigonometric functions sine, cosine, and tangent are used to create 3D models and simulate real-world environments.
In computer graphics, trigonometry is used to calculate the position and orientation of objects in 3D space. This information is used to create realistic lighting effects, simulate motion, and render 3D models.
**

4. Medical Imaging
Trigonometry is used in medical imaging techniques such as MRI and CT scans. The trigonometric functions sine and cosine are used to reconstruct images of the body from raw data.
In MRI scans, trigonometry is used to calculate the position and orientation of hydrogen atoms in the body. This information is used to create detailed images of internal organs and tissues.
**

5. Music and Acoustics
Trigonometry is used in music and acoustics to analyze and synthesize sound waves. The trigonometric functions sine and cosine are used to describe the behavior of sound waves, including their frequency, amplitude, and phase.
In music synthesis, trigonometry is used to create realistic sound effects and simulate the behavior of musical instruments.
**

Gallery of Trigonometry in Real-Life Applications





Frequently Asked Questions
What is trigonometry used for in real-life applications?
+Trigonometry is used in various real-life applications, including navigation, physics, engineering, computer science, medical imaging, and music.
How is trigonometry used in navigation?
+Trigonometry is used in navigation to calculate distances and angles between landmarks and reference points. It is also used in GPS systems to determine the location of a vehicle or device.
What is the role of trigonometry in medical imaging?
+Trigonometry is used in medical imaging techniques such as MRI and CT scans to reconstruct images of the body from raw data.
In conclusion, trigonometry is a vital branch of mathematics that has numerous practical applications in various industries. From navigation and physics to computer science and medical imaging, trigonometry plays a crucial role in solving complex problems and making our lives easier.