Rational numbers have been a cornerstone of mathematics for centuries, providing a fundamental framework for understanding various mathematical concepts. One of the key aspects of rational numbers is that they form a basis for various mathematical structures, allowing us to build upon them to explore more complex ideas. In this article, we will delve into five ways rational numbers form a basis for mathematical constructs, highlighting their significance in the realm of mathematics.
What are Rational Numbers?
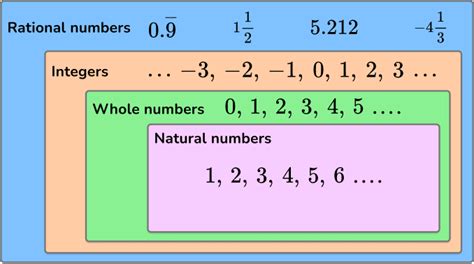
Before we dive into the ways rational numbers form a basis, it is essential to understand what rational numbers are. Rational numbers are numbers that can be expressed as the ratio of two integers, where the denominator is non-zero. This means that any number that can be written in the form a/b, where a and b are integers and b ≠ 0, is a rational number. Examples of rational numbers include 1/2, 3/4, and 22/7.

1. Basis for Real Numbers
One of the most significant ways rational numbers form a basis is for real numbers. Real numbers include all rational numbers, as well as irrational numbers, which cannot be expressed as a finite decimal or fraction. Rational numbers provide a foundation for real numbers, allowing us to construct the real number line. The real number line is a fundamental concept in mathematics, enabling us to visualize and work with various mathematical concepts, such as algebra and calculus.
How Rational Numbers Form a Basis for Real Numbers
Rational numbers form a basis for real numbers by providing a set of numbers that can be used to construct the real number line. The real number line is constructed by adding rational numbers to the set of integers, allowing us to create a continuous line that includes all rational and irrational numbers. This construction is essential for various mathematical applications, including geometry, trigonometry, and calculus.

2. Basis for Algebraic Structures
Rational numbers also form a basis for various algebraic structures, such as groups, rings, and fields. These structures are essential for abstract algebra, allowing us to study the properties and behavior of algebraic objects. Rational numbers provide a foundation for these structures, enabling us to construct and analyze various algebraic objects, such as polynomials, matrices, and vector spaces.
How Rational Numbers Form a Basis for Algebraic Structures
Rational numbers form a basis for algebraic structures by providing a set of numbers that can be used to construct and analyze algebraic objects. For example, rational numbers can be used to construct polynomial rings, which are essential for studying polynomial equations and algebraic geometry. Rational numbers also provide a foundation for matrix theory, allowing us to construct and analyze matrices, which are crucial for linear algebra and its applications.

3. Basis for Mathematical Analysis
Rational numbers form a basis for mathematical analysis, including calculus, differential equations, and mathematical physics. Rational numbers provide a foundation for mathematical analysis by allowing us to construct and analyze mathematical functions, which are essential for modeling real-world phenomena.
How Rational Numbers Form a Basis for Mathematical Analysis
Rational numbers form a basis for mathematical analysis by providing a set of numbers that can be used to construct and analyze mathematical functions. For example, rational numbers can be used to construct polynomial functions, which are essential for studying polynomial equations and algebraic geometry. Rational numbers also provide a foundation for trigonometric functions, which are crucial for modeling periodic phenomena in physics and engineering.

4. Basis for Geometry
Rational numbers form a basis for geometry, allowing us to construct and analyze geometric objects, such as points, lines, and planes. Rational numbers provide a foundation for geometry by enabling us to define and work with geometric coordinates, which are essential for studying geometric shapes and their properties.
How Rational Numbers Form a Basis for Geometry
Rational numbers form a basis for geometry by providing a set of numbers that can be used to construct and analyze geometric objects. For example, rational numbers can be used to construct coordinate systems, which are essential for studying geometric shapes and their properties. Rational numbers also provide a foundation for geometric transformations, which are crucial for studying symmetries and geometric invariants.

5. Basis for Computer Science
Rational numbers form a basis for computer science, allowing us to construct and analyze algorithms, data structures, and software systems. Rational numbers provide a foundation for computer science by enabling us to define and work with computational models, which are essential for studying computational complexity and algorithmic efficiency.
How Rational Numbers Form a Basis for Computer Science
Rational numbers form a basis for computer science by providing a set of numbers that can be used to construct and analyze computational models. For example, rational numbers can be used to construct numerical algorithms, which are essential for solving computational problems in science, engineering, and finance. Rational numbers also provide a foundation for data structures, which are crucial for storing and retrieving data in computer systems.







What is a rational number?
+A rational number is a number that can be expressed as the ratio of two integers, where the denominator is non-zero.
How do rational numbers form a basis for real numbers?
+Rational numbers form a basis for real numbers by providing a set of numbers that can be used to construct the real number line.
What is the significance of rational numbers in mathematics?
+Rational numbers are significant in mathematics because they provide a foundation for various mathematical structures, including real numbers, algebraic structures, and geometric objects.
In conclusion, rational numbers form a basis for various mathematical structures, including real numbers, algebraic structures, geometric objects, mathematical analysis, and computer science. Their significance in mathematics cannot be overstated, as they provide a foundation for understanding and working with various mathematical concepts. We hope this article has provided you with a deeper understanding of the importance of rational numbers in mathematics.