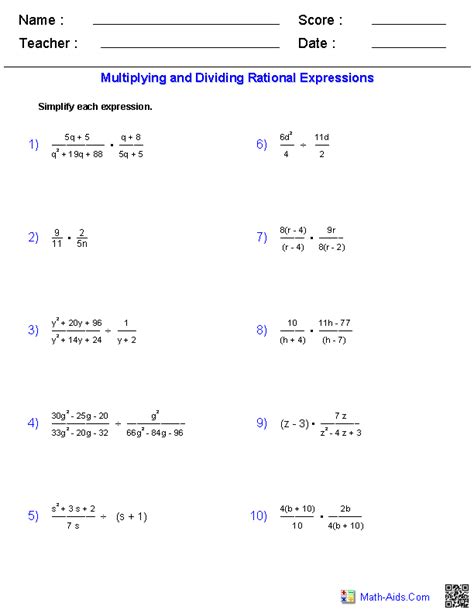
Rational expressions are a fundamental concept in algebra, and understanding their applications is crucial for problem-solving in various fields, including physics, engineering, and economics. In this article, we will delve into the world of rational expressions, exploring their definitions, types, and applications. We will also provide a comprehensive answer key to a worksheet on rational expressions, explaining each solution in detail.
What are Rational Expressions?
A rational expression is a fraction that contains one or more variables in the numerator, denominator, or both. It is a quotient of two polynomials, where the numerator and denominator are separated by a horizontal line or a forward slash (/). Rational expressions can be simplified, added, subtracted, multiplied, and divided, just like numerical fractions.
Types of Rational Expressions
There are several types of rational expressions, including:
- Proper Rational Expressions: The degree of the numerator is less than the degree of the denominator.
- Improper Rational Expressions: The degree of the numerator is greater than or equal to the degree of the denominator.
- Mixed Rational Expressions: A combination of a polynomial and a rational expression.
Simplifying Rational Expressions
Simplifying rational expressions involves canceling out common factors between the numerator and denominator. This process is crucial in solving rational expression problems. To simplify a rational expression, follow these steps:
- Factor the numerator and denominator.
- Cancel out common factors.
- Rewrite the expression in its simplest form.
Applications of Rational Expressions
Rational expressions have numerous applications in various fields, including:
- Physics and Engineering: Rational expressions are used to model real-world phenomena, such as electrical circuits, mechanical systems, and optics.
- Economics: Rational expressions are used to model economic systems, including supply and demand curves.
- Computer Science: Rational expressions are used in algorithms for solving systems of linear equations.
Rational Expressions Applications Worksheet Answer Key
Now, let's dive into the answer key for a worksheet on rational expressions applications.
Problem 1
Simplify the rational expression: (x^2 + 3x) / (x^2 - 4)
Solution
Factor the numerator and denominator: (x^2 + 3x) = x(x + 3) (x^2 - 4) = (x + 2)(x - 2)
Cancel out common factors: x(x + 3) / (x + 2)(x - 2) = (x + 3) / (x - 2)
Rewrite the expression in its simplest form: (x + 3) / (x - 2)
Problem 2
Solve the equation: (2x + 1) / (x - 2) = 3 / 2
Solution
Cross-multiply: 2(2x + 1) = 3(x - 2)
Expand and simplify: 4x + 2 = 3x - 6
Subtract 3x from both sides: x + 2 = -6
Subtract 2 from both sides: x = -8
Problem 3
Simplify the rational expression: (x^2 - 4) / (x^2 + 3x)
Solution
Factor the numerator and denominator: (x^2 - 4) = (x + 2)(x - 2) (x^2 + 3x) = x(x + 3)
Cancel out common factors: (x + 2)(x - 2) / x(x + 3) = (x - 2) / (x + 3)
Rewrite the expression in its simplest form: (x - 2) / (x + 3)
Image

Gallery of Rational Expressions Applications





FAQ Section
What is a rational expression?
+A rational expression is a fraction that contains one or more variables in the numerator, denominator, or both.
How do I simplify a rational expression?
+To simplify a rational expression, factor the numerator and denominator, cancel out common factors, and rewrite the expression in its simplest form.
What are some applications of rational expressions?
+Rational expressions have numerous applications in various fields, including physics, engineering, economics, and computer science.
We hope this comprehensive article has provided you with a deeper understanding of rational expressions and their applications. If you have any questions or need further clarification, please don't hesitate to ask. Share your thoughts and feedback in the comments section below!