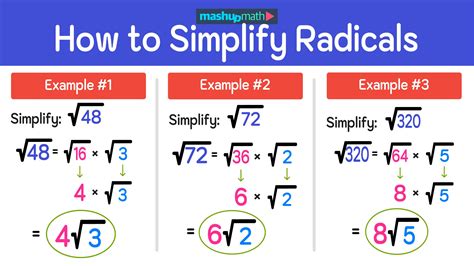
Simplifying radical expressions is a crucial skill in mathematics, particularly in algebra and geometry. Radical expressions, also known as roots, can be intimidating at first, but breaking them down into manageable steps can make the process much more accessible. In this article, we will explore the three easy steps to simplify radical expressions, making it easier for you to tackle complex math problems.
Why Simplify Radical Expressions?
Before diving into the steps, it's essential to understand why simplifying radical expressions is necessary. Simplifying radicals helps to:
- Make calculations easier
- Reduce errors
- Enhance understanding of mathematical concepts
- Improve problem-solving skills
Step 1: Factor Out Perfect Squares
The first step in simplifying radical expressions is to factor out perfect squares. A perfect square is a number that can be expressed as the square of an integer, such as 4, 9, or 16.

For example, let's simplify the radical expression √(16x^2). We can factor out the perfect square 16, which is equal to 4^2. This gives us:
√(16x^2) = √(4^2 x^2) = 4x
Step 2: Simplify the Radical
Once you've factored out perfect squares, the next step is to simplify the radical. This involves reducing the radical to its simplest form.

For instance, let's simplify the radical expression √(8x). We can break down 8 into its prime factors, which are 2 x 2 x 2. This gives us:
√(8x) = √(2 x 2 x 2 x) = 2√(2x)
Step 3: Combine Like Terms
The final step in simplifying radical expressions is to combine like terms. This involves adding or subtracting like terms, such as 2x and 3x.

For example, let's simplify the radical expression √(2x) + √(3x). We can combine the like terms:
√(2x) + √(3x) = √(5x)
Gallery of Simplifying Radical Expressions






Frequently Asked Questions
What is a radical expression?
+A radical expression is a mathematical expression that contains a root, such as a square root or cube root.
Why do I need to simplify radical expressions?
+Simplifying radical expressions makes calculations easier, reduces errors, and enhances understanding of mathematical concepts.
How do I simplify a radical expression?
+To simplify a radical expression, factor out perfect squares, simplify the radical, and combine like terms.
In conclusion, simplifying radical expressions is a straightforward process that involves factoring out perfect squares, simplifying the radical, and combining like terms. By following these three easy steps, you can tackle complex math problems with confidence. Remember to practice regularly to reinforce your understanding of radical expressions and to become proficient in simplifying them.