The world of mechanics and engineering is full of complex calculations and conversions, but with the right tools and knowledge, even the most daunting tasks can become manageable. One such conversion that is commonly encountered in the field of mechanics is the conversion of radians to revolutions per minute (RPM). In this article, we will explore the importance of this conversion, the formula used to perform it, and provide step-by-step instructions on how to make this conversion with ease.
Understanding Radians and RPM
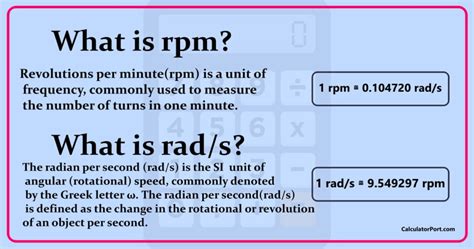
Before we dive into the conversion process, it's essential to understand the two units involved. Radians are a unit of angular measurement, representing the ratio of the arc length to the radius of a circle. On the other hand, RPM is a unit of rotational speed, measuring the number of complete rotations or revolutions per minute.

Why is Radians to RPM Conversion Important?
The conversion of radians to RPM is crucial in various fields, including engineering, mechanics, and physics. This conversion is necessary when dealing with rotational motion, as it allows us to express the speed of an object in a more meaningful and relatable way. For instance, when designing a motor or a gear system, engineers need to convert the angular velocity from radians to RPM to ensure the system operates within the desired parameters.

The Formula for Radians to RPM Conversion
The formula for converting radians to RPM is:
RPM = (Radians × 60) / (2π)
Where:
- RPM is the rotational speed in revolutions per minute
- Radians is the angular velocity in radians per second
- π is the mathematical constant representing the ratio of a circle's circumference to its diameter (approximately 3.14)
Step-by-Step Instructions for Radians to RPM Conversion
Now that we have the formula, let's go through the step-by-step process of converting radians to RPM:
- Convert the angular velocity from radians to revolutions: Divide the angular velocity in radians by 2π to get the number of revolutions.
- Multiply the result by 60: Multiply the number of revolutions by 60 to convert the time from seconds to minutes.
- Perform the calculation: Plug in the values into the formula and perform the calculation to get the RPM value.
Example Calculations
To illustrate the conversion process, let's consider a few examples:
- Example 1: Convert 10 radians per second to RPM
RPM = (10 × 60) / (2π) ≈ 95.5 RPM
- Example 2: Convert 20 radians per second to RPM
RPM = (20 × 60) / (2π) ≈ 191 RPM
- Example 3: Convert 50 radians per second to RPM
RPM = (50 × 60) / (2π) ≈ 477.5 RPM

Practical Applications of Radians to RPM Conversion
The conversion of radians to RPM has numerous practical applications in various fields, including:
- Mechanical engineering: When designing mechanical systems, such as gearboxes or motors, engineers need to convert the angular velocity from radians to RPM to ensure the system operates within the desired parameters.
- Aerospace engineering: In aerospace engineering, the conversion of radians to RPM is crucial for calculating the speed of aircraft and spacecraft.
- Physics and mathematics: The conversion of radians to RPM is used in various physics and mathematics problems, such as calculating the speed of rotating objects or the frequency of oscillating systems.

Conclusion
In conclusion, the conversion of radians to RPM is an essential calculation in various fields, including engineering, mechanics, and physics. By understanding the formula and following the step-by-step instructions, you can easily make this conversion and ensure accurate results. Whether you're a student, engineer, or simply someone interested in mechanics, this conversion is a valuable tool to have in your toolkit.
Gallery of Radians to RPM Conversion






FAQs
What is the formula for converting radians to RPM?
+The formula for converting radians to RPM is: RPM = (Radians × 60) / (2π)
Why is the conversion of radians to RPM important?
+The conversion of radians to RPM is crucial in various fields, including engineering, mechanics, and physics, as it allows us to express the speed of an object in a more meaningful and relatable way.
What are some practical applications of radians to RPM conversion?
+The conversion of radians to RPM has numerous practical applications, including mechanical engineering, aerospace engineering, and physics and mathematics problems.