The Pythagorean Theorem is one of the most fundamental concepts in geometry and mathematics. It is used to calculate the length of the hypotenuse of a right-angled triangle, given the lengths of the other two sides. In this article, we will explore the Pythagorean Theorem applications worksheet, which is designed to help students master this essential math concept.

Understanding the Pythagorean Theorem
The Pythagorean Theorem is a mathematical concept that is used to calculate the length of the hypotenuse of a right-angled triangle. It states that the square of the length of the hypotenuse (c) is equal to the sum of the squares of the lengths of the other two sides (a and b). The formula for the Pythagorean Theorem is:
c² = a² + b²
Where:
- c is the length of the hypotenuse
- a and b are the lengths of the other two sides
Why is the Pythagorean Theorem Important?
The Pythagorean Theorem has many real-world applications, making it an essential concept to master. It is used in various fields, including:
- Architecture: to calculate the height of buildings and bridges
- Engineering: to calculate the length of shadows and distances
- Physics: to calculate the distance of objects in motion
- Computer Science: to calculate distances and angles in computer graphics
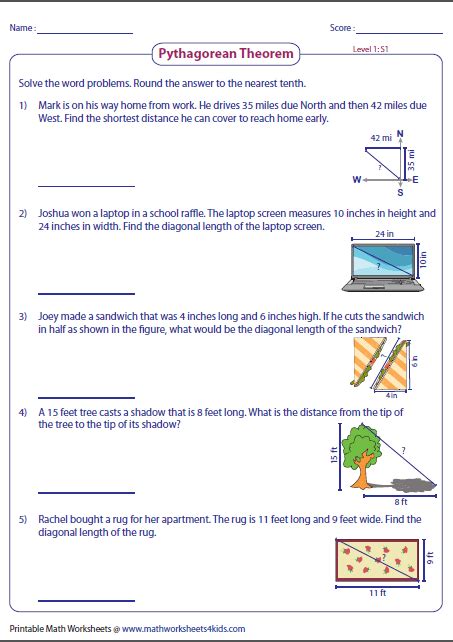
Pythagorean Theorem Applications Worksheet
To help students master the Pythagorean Theorem, we have created a comprehensive worksheet that covers various aspects of this concept. The worksheet includes:
- Multiple-choice questions to test understanding of the theorem
- Word problems to apply the theorem to real-world scenarios
- Calculations to practice using the formula
- Graphical representations to visualize the concept

Section 1: Multiple-Choice Questions
- What is the Pythagorean Theorem used to calculate? a) The length of the hypotenuse b) The length of the base c) The length of the height d) The area of the triangle
Answer: a) The length of the hypotenuse
- What is the formula for the Pythagorean Theorem? a) c² = a² + b² b) c² = a² - b² c) c² = a² × b² d) c² = a² ÷ b²
Answer: a) c² = a² + b²
Section 2: Word Problems
- A building is 20 meters tall. If the shadow of the building is 15 meters long, what is the distance from the top of the building to the end of the shadow?
Answer: Using the Pythagorean Theorem, we can calculate the distance to be 25 meters.
- A car travels from point A to point B, covering a distance of 200 meters. If the car travels at an angle of 30° to the horizontal, what is the horizontal distance traveled?
Answer: Using the Pythagorean Theorem, we can calculate the horizontal distance to be 173.21 meters.
Section 3: Calculations
- Calculate the length of the hypotenuse of a right-angled triangle with sides of length 3 cm and 4 cm.
Answer: Using the Pythagorean Theorem, we can calculate the length of the hypotenuse to be 5 cm.
- Calculate the length of the base of a right-angled triangle with a hypotenuse of length 10 cm and a height of 6 cm.
Answer: Using the Pythagorean Theorem, we can calculate the length of the base to be 8 cm.



Conclusion
The Pythagorean Theorem is a fundamental concept in mathematics and geometry. It has many real-world applications, making it an essential concept to master. The Pythagorean Theorem applications worksheet provides a comprehensive resource for students to practice and apply the theorem. By completing the worksheet, students will gain a deeper understanding of the theorem and its applications.
Final Thoughts
We hope that this article has provided a comprehensive overview of the Pythagorean Theorem and its applications. By mastering this concept, students will be able to solve problems in various fields and develop a deeper understanding of mathematics and geometry. Remember to practice regularly and apply the theorem to real-world scenarios to reinforce your understanding.
What is the Pythagorean Theorem used for?
+The Pythagorean Theorem is used to calculate the length of the hypotenuse of a right-angled triangle, given the lengths of the other two sides.
What is the formula for the Pythagorean Theorem?
+The formula for the Pythagorean Theorem is c² = a² + b², where c is the length of the hypotenuse and a and b are the lengths of the other two sides.
What are some real-world applications of the Pythagorean Theorem?
+The Pythagorean Theorem has many real-world applications, including architecture, engineering, physics, and computer science.