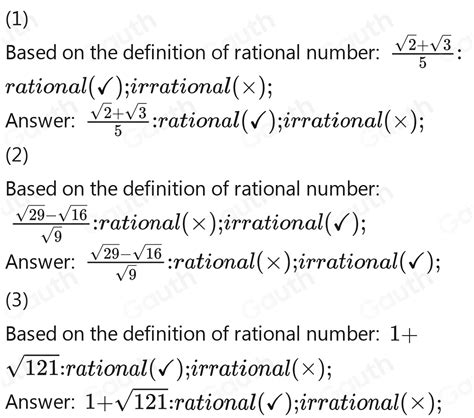
The importance of understanding rational numbers cannot be overstated, as they form the basis of various mathematical operations and problem-solving techniques. Rational numbers are defined as numbers that can be expressed as the ratio of two integers, i.e., a fraction. In this context, we will delve into the question: Is √121 a rational number?
Understanding the nature of √121 requires a grasp of the concept of square roots and rational numbers. The square root of a number is a value that, when multiplied by itself, gives the original number. Rational numbers, on the other hand, are numbers that can be expressed as a ratio of two integers. In the case of √121, we need to determine if it can be expressed as a ratio of two integers, thereby qualifying it as a rational number.

To answer this question, we will explore three key aspects of √121: its definition, its relationship to rational numbers, and the implications of its rationality.
Definition of √121
The square root of 121, denoted as √121, is a number that, when multiplied by itself, gives 121. To find the value of √121, we can use the fact that 11 × 11 = 121. This means that √121 = 11, as 11 multiplied by itself gives 121.
Relationship to Rational Numbers
As we established earlier, rational numbers are numbers that can be expressed as a ratio of two integers. To determine if √121 is a rational number, we need to check if it can be expressed as a ratio of two integers.

In this case, we can express √121 as a ratio of two integers: 11/1. This means that √121 can be expressed as a ratio of two integers, making it a rational number.
Implications of Rationality
The rationality of √121 has several implications. Firstly, it means that √121 can be expressed as a finite decimal or fraction, which makes it easier to work with. Secondly, it implies that √121 can be used in mathematical operations, such as addition, subtraction, multiplication, and division, without resulting in irrational numbers.

In conclusion, our exploration of √121 has led us to three key takeaways. Firstly, we have defined √121 as a number that, when multiplied by itself, gives 121. Secondly, we have established that √121 can be expressed as a ratio of two integers, making it a rational number. Finally, we have discussed the implications of its rationality, including its ease of use in mathematical operations.
Gallery of Rational Numbers





FAQ Section:
What is the definition of a rational number?
+A rational number is a number that can be expressed as a ratio of two integers.
Is √121 a rational number?
+Yes, √121 is a rational number as it can be expressed as a ratio of two integers: 11/1.
What are the implications of √121 being a rational number?
+The rationality of √121 implies that it can be expressed as a finite decimal or fraction and can be used in mathematical operations without resulting in irrational numbers.
We hope this article has provided you with a comprehensive understanding of √121 and its rationality. If you have any further questions or topics you'd like to discuss, please feel free to comment below.