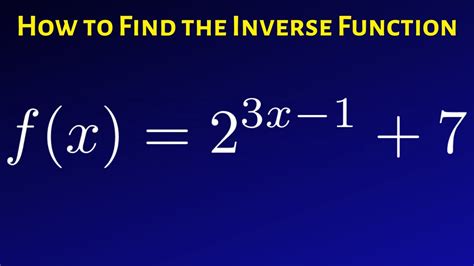
The concept of inverse functions is a fundamental idea in mathematics, and it has numerous applications in various fields such as physics, engineering, and economics. When it comes to exponential functions, finding their inverses can be a bit challenging, but there are several techniques that can help. In this article, we will explore five ways to find the inverse of exponential functions.
Exponential functions are widely used to model population growth, chemical reactions, and other phenomena where the rate of change is proportional to the current value. The general form of an exponential function is f(x) = ab^x, where a and b are constants, and x is the independent variable. Finding the inverse of an exponential function involves finding a new function that will "undo" the original function.
Method 1: Using the Definition of Inverse Functions
One way to find the inverse of an exponential function is to use the definition of inverse functions. The inverse of a function f(x) is denoted by f^(-1)(x), and it is defined as a function that satisfies the following condition:
f(f^(-1)(x)) = x
To find the inverse of an exponential function f(x) = ab^x, we can start by writing the equation y = ab^x and then solving for x in terms of y.

This method involves some algebraic manipulations, but it provides a general approach for finding the inverse of any exponential function.
Step-by-Step Procedure
- Write the equation y = ab^x.
- Take the logarithm of both sides of the equation.
- Use the properties of logarithms to simplify the equation.
- Solve for x in terms of y.
Method 2: Using Logarithmic Functions
Another way to find the inverse of an exponential function is to use logarithmic functions. The logarithmic function is the inverse of the exponential function, and it can be used to solve equations involving exponential functions.
The logarithmic function is defined as:
log_b(x) = y ⇔ b^y = x
To find the inverse of an exponential function f(x) = ab^x, we can use the logarithmic function to solve for x in terms of y.

This method is based on the fact that the logarithmic function is the inverse of the exponential function, and it provides a straightforward way to find the inverse of exponential functions.
Step-by-Step Procedure
- Write the equation y = ab^x.
- Take the logarithm of both sides of the equation.
- Use the properties of logarithms to simplify the equation.
- Solve for x in terms of y.
Method 3: Using Graphical Analysis
Graphical analysis is another way to find the inverse of an exponential function. By plotting the graph of the exponential function and reflecting it about the line y = x, we can obtain the graph of the inverse function.
This method is based on the fact that the graph of the inverse function is the reflection of the graph of the original function about the line y = x.

This method provides a visual approach to finding the inverse of exponential functions and can be useful for understanding the relationship between the original function and its inverse.
Method 4: Using the Inverse Function Theorem
The inverse function theorem is a mathematical result that provides a sufficient condition for a function to have an inverse. The theorem states that if a function f(x) is continuous and one-to-one on an interval, then it has an inverse function on that interval.
To find the inverse of an exponential function f(x) = ab^x, we can use the inverse function theorem to show that the function is one-to-one and then find its inverse using the definition of inverse functions.

This method provides a rigorous approach to finding the inverse of exponential functions and can be useful for understanding the mathematical properties of inverse functions.
Method 5: Using Algebraic Manipulations
Finally, we can use algebraic manipulations to find the inverse of an exponential function. By manipulating the equation y = ab^x, we can solve for x in terms of y and obtain the inverse function.
This method involves using algebraic techniques such as substitution, elimination, and rearrangement to solve for x in terms of y.

This method provides a flexible approach to finding the inverse of exponential functions and can be useful for understanding the algebraic structure of inverse functions.
Gallery of Inverse Functions





Frequently Asked Questions
What is the definition of an inverse function?
+An inverse function is a function that undoes the action of another function.
How do I find the inverse of an exponential function?
+There are several ways to find the inverse of an exponential function, including using the definition of inverse functions, logarithmic functions, graphical analysis, the inverse function theorem, and algebraic manipulations.
What is the relationship between exponential functions and logarithmic functions?
+Exponential functions and logarithmic functions are inverse functions, meaning that they undo each other.
In conclusion, finding the inverse of an exponential function is an important concept in mathematics, and there are several ways to approach it. By using the definition of inverse functions, logarithmic functions, graphical analysis, the inverse function theorem, and algebraic manipulations, we can find the inverse of exponential functions and understand their properties.