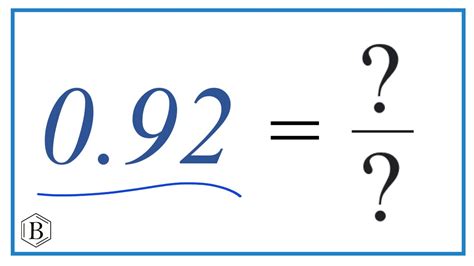In the vast world of mathematics, fractions play a crucial role in representing parts of a whole. One such fraction that sparks curiosity is 0.38 as a fraction simplified.
Imagine you're a budding mathematician, and you stumble upon the decimal 0.38. Your curiosity is piqued, and you wonder what this decimal would look like in its fraction form. You start pondering the possibilities, and before you know it, you're on a mission to simplify 0.38 into a fraction.
Understanding Decimals and Fractions
To tackle this problem, let's first understand the relationship between decimals and fractions. Decimals are a way of expressing numbers with a fractional part, whereas fractions represent a part of a whole. The key to converting decimals to fractions lies in identifying the place value of the decimal.
In the case of 0.38, we have a decimal with two digits after the decimal point. This means we can express 0.38 as a fraction with a denominator of 100, since there are two decimal places.
Converting 0.38 to a Fraction
Now that we've established the denominator, let's convert 0.38 to a fraction. We can write 0.38 as:
0.38 = 38/100
To simplify this fraction, we need to find the greatest common divisor (GCD) of 38 and 100.
Simplifying the Fraction
The GCD of 38 and 100 is 2. To simplify the fraction, we divide both the numerator and denominator by 2:
38 ÷ 2 = 19 100 ÷ 2 = 50
So, the simplified fraction for 0.38 is:
0.38 = 19/50

Real-World Applications
Now that we've simplified 0.38 as a fraction, let's explore some real-world applications of this concept.
In cooking, you might need to measure ingredients with precision. Converting decimals to fractions can help you achieve the perfect recipe. For instance, if a recipe calls for 0.38 cups of sugar, you can use the simplified fraction 19/50 to measure it accurately.
In finance, decimals and fractions are crucial in calculating interest rates, investment returns, and taxes. Understanding how to convert decimals to fractions can help you make informed decisions about your financial future.
Practical Examples
Here are a few more examples to illustrate the concept:
- 0.25 as a fraction is 1/4
- 0.75 as a fraction is 3/4
- 0.125 as a fraction is 1/8
These examples demonstrate how decimals can be converted to fractions and simplified for easy use in various applications.

Conclusion and Final Thoughts
In conclusion, converting decimals to fractions is an essential math concept with numerous real-world applications. By understanding how to simplify decimals, you can improve your problem-solving skills and make more accurate calculations.
Whether you're a math enthusiast or just starting to explore the world of fractions, remember that practice makes perfect. Try converting different decimals to fractions and simplifying them to become more confident in your math abilities.

What's Next?
We'd love to hear from you! Share your thoughts on decimals and fractions in the comments below. If you have any questions or topics you'd like us to explore, feel free to ask.
Before we bid adieu, take a moment to appreciate the beauty of fractions and decimals. These mathematical concepts might seem simple, but they hold the key to unlocking a world of possibilities.
Stay curious, keep exploring, and remember: math is all around us!
Gallery of Fractions and Decimals






What is the difference between a decimal and a fraction?
+A decimal is a way of expressing numbers with a fractional part, whereas a fraction represents a part of a whole.
How do I convert a decimal to a fraction?
+To convert a decimal to a fraction, identify the place value of the decimal and express it as a fraction with the corresponding denominator.
What is the greatest common divisor (GCD) of two numbers?
+The GCD of two numbers is the largest number that divides both numbers without leaving a remainder.