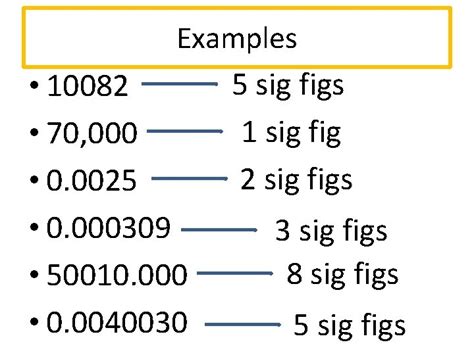
Mastering 1.20: 3 Essential Rules For Sig Figs

Quick Links :

Mastering significant figures (sig figs) is a crucial skill for anyone working with numerical data, whether in science, engineering, or finance. Sig figs are a way to express the precision of a measurement or calculation, and understanding the rules that govern them is essential for maintaining accuracy and avoiding errors. In this article, we will explore the three essential rules for sig figs and provide practical examples to help you master this important concept.

The Importance of Sig Figs

Sig figs are a shorthand way to indicate the number of significant digits in a numerical value. Significant digits are the digits in a number that are known to be reliable and certain, and they are used to express the precision of a measurement or calculation. For example, the number 123.45 has five significant digits, while the number 0.0012345 has six significant digits.

Understanding sig figs is important because it helps you to:

• Express the precision of a measurement or calculation
• Avoid rounding errors and maintain accuracy
• Communicate numerical data clearly and unambiguously

Rule 1: Non-Zero Digits Are Always Significant

The first rule of sig figs states that non-zero digits are always significant. This means that any digit that is not zero is considered to be a significant digit. For example:

• 123.45 has five significant digits (1, 2, 3, 4, and 5)
• 0.0012345 has six significant digits (1, 2, 3, 4, and 5)

This rule is straightforward and easy to apply, but it's essential to remember that non-zero digits are always significant, regardless of their position in the number.

Rule 2: Zeros Between Non-Zero Digits Are Significant

The second rule of sig figs states that zeros between non-zero digits are significant. This means that any zero that is sandwiched between two non-zero digits is considered to be a significant digit. For example:

• 120.45 has five significant digits (1, 2, 0, 4, and 5)
• 0.0010230 has seven significant digits (0, 0, 1, 0, 2, 3, and 0)

This rule can be a bit tricky to apply, but it's essential to remember that zeros between non-zero digits are always significant.

Rule 3: Trailing Zeros Are Significant If the Number Contains a Decimal Point

The third rule of sig figs states that trailing zeros are significant if the number contains a decimal point. This means that any zero that is located at the end of a number and is preceded by a decimal point is considered to be a significant digit. For example:

• 12.450 has five significant digits (1, 2, 4, 5, and 0)
• 0.00123450 has seven significant digits (0, 0, 1, 2, 3, 4, and 5)

This rule can be a bit nuanced to apply, but it's essential to remember that trailing zeros are significant if the number contains a decimal point.

Practical Examples of Sig Figs

To illustrate the application of these rules, let's consider a few practical examples:

• Measuring the length of a room: If you measure the length of a room to be 12.45 meters, you would express this value as 12.45 meters, with four significant digits (1, 2, 4, and 5).
• Calculating the area of a rectangle: If you calculate the area of a rectangle to be 0.0012345 square meters, you would express this value as 0.0012345 square meters, with six significant digits (1, 2, 3, 4, and 5).