Convert 0.8 Repeating To A Fraction In 3 Easy Steps

Quick Links :

Converting a repeating decimal to a fraction can seem daunting, but it's actually a straightforward process. Let's take the example of 0.8 repeating, which we'll convert to a fraction in three easy steps.

Understanding the Problem

0.8 repeating, also written as 0.888..., represents a decimal that goes on indefinitely with the digit 8 repeating. To convert this decimal to a fraction, we need to identify the repeating pattern and use it to set up an equation.

Step 1: Identify the Repeating Pattern

In this case, the repeating pattern is simply the digit 8. We can represent 0.8 repeating as x = 0.888...

Step 2: Multiply the Equation by a Power of 10

To eliminate the repeating decimal, we'll multiply both sides of the equation by a power of 10. Since the repeating pattern is only one digit long, we'll multiply by 10.

10x = 8.888...

This multiplication shifts the decimal point one place to the right, which allows us to subtract the original equation from this new equation.

Step 3: Subtract the Original Equation

Now, we'll subtract the original equation from the new equation to eliminate the repeating decimal.

10x - x = 8.888... - 0.888... 9x = 8

Dividing both sides by 9, we get:

x = 8/9

And there you have it! 0.8 repeating converted to a fraction is 8/9.

In this example, we've seen how to convert a repeating decimal to a fraction using a simple three-step process. This technique can be applied to any repeating decimal, as long as you identify the repeating pattern and adjust the multiplication factor accordingly.

Other Examples

Let's take a look at a few more examples of converting repeating decimals to fractions.

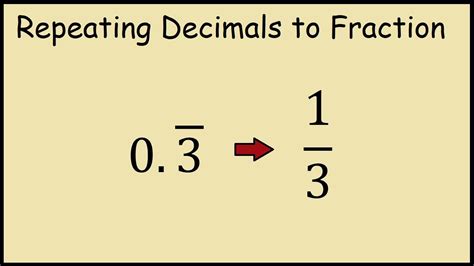
• 0.3 repeating = 3/9 = 1/3
• 0.6 repeating = 6/9 = 2/3
• 0.9 repeating = 9/9 = 1

As you can see, the process is the same, and the only difference is the length of the repeating pattern and the multiplication factor.

Benefits of Converting Repeating Decimals to Fractions

Converting repeating decimals to fractions can have several benefits. For one, it allows us to express the number in a more compact and elegant form. Additionally, fractions can be easier to work with in mathematical calculations, especially when dealing with proportions and ratios.

Common Mistakes

When converting repeating decimals to fractions, there are a few common mistakes to watch out for.

• Make sure to identify the correct repeating pattern and adjust the multiplication factor accordingly.
• Avoid subtracting the wrong equations or multiplying by the wrong power of 10.
• Double-check your work to ensure that the resulting fraction is in simplest form.

By following these steps and avoiding common mistakes, you can confidently convert repeating decimals to fractions and improve your math skills.

Practical Applications

Converting repeating decimals to fractions has numerous practical applications in various fields, including:

• Mathematics

Converting repeating decimals to fractions is an essential skill in mathematics, particularly in algebra, geometry, and trigonometry.

• Science

In science, fractions are often used to express measurements and proportions. Converting repeating decimals to fractions can help scientists and engineers make more accurate calculations.

• Finance

In finance, fractions are used to express interest rates, investment returns, and other financial metrics. Converting repeating decimals to fractions can help financial professionals make more informed decisions.

In conclusion, converting 0.8 repeating to a fraction is a simple process that can be accomplished in three easy steps. By following these steps and avoiding common mistakes, you can improve your math skills and apply this technique to a wide range of practical problems.

Gallery of Printable Conversion Tables

Frequently Asked Questions