Convert 0.3 Repeating To A Fraction

Quick Links :

Hide

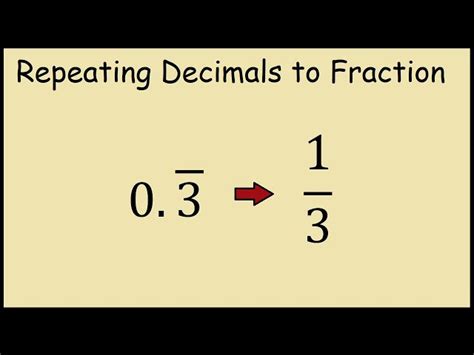
Understanding 0.3 Repeating

The decimal 0.3 repeating is a common mathematical representation that can be easily converted to a fraction. In this article, we'll explore the steps to convert 0.3 repeating to a fraction and understand the underlying mathematical concepts.

What is 0.3 Repeating?

0.3 repeating is a decimal representation where the digit 3 repeats infinitely. It can be written as 0.333..., where the dots indicate that the digit 3 continues to repeat without end.

Converting 0.3 Repeating to a Fraction

To convert 0.3 repeating to a fraction, we can use a simple algebraic method. Let's denote 0.3 repeating as x.

x = 0.333...

We can multiply both sides of the equation by 10 to get:

10x = 3.333...

Now, subtract the original equation from the new equation:

10x - x = 3.333... - 0.333...

This simplifies to:

9x = 3

Divide both sides by 9:

x = 3/9

x = 1/3

Therefore, 0.3 repeating is equal to the fraction 1/3.

Why Does This Method Work?

This method works because multiplying both sides of the equation by 10 shifts the decimal point one place to the right, which allows us to subtract the original equation and eliminate the repeating decimal. The resulting equation is a simple linear equation that can be solved for x.

Other Ways to Convert 0.3 Repeating to a Fraction

There are other methods to convert 0.3 repeating to a fraction, including:

• Using a calculator or online conversion tool
• Using a mathematical software or programming language
• Using a formula or algorithm specifically designed for converting repeating decimals to fractions

However, the algebraic method presented above is a simple and effective way to convert 0.3 repeating to a fraction.

Real-World Applications

Converting 0.3 repeating to a fraction has many real-world applications, including:

• Calculating interest rates and investment returns
• Determining proportions and ratios in science and engineering
• Simplifying complex mathematical expressions and equations

In conclusion, converting 0.3 repeating to a fraction is a simple and important mathematical concept that has many practical applications. By using the algebraic method presented above, we can easily convert 0.3 repeating to the fraction 1/3.

Gallery of Converting Decimals to Fractions

We hope this article has been informative and helpful in understanding how to convert 0.3 repeating to a fraction. If you have any further questions or comments, please feel free to share them below.